Unlocking The Secrets Of Standard Form Polynomial Expression

louisamayalcott

29 Apr, 2025

Hey there math enthusiasts! Get ready to dive deep into the fascinating world of standard form polynomial expressions. If you're scratching your head right now, don't worry, I've got you covered. This isn't just another boring math lesson; it's your gateway to mastering one of the most essential concepts in algebra. So, buckle up and let's get started, shall we?

Standard form polynomial expression might sound intimidating, but it's actually pretty straightforward once you get the hang of it. Think of it as organizing your math equations in a neat and orderly fashion. It's like cleaning up your room, but instead of clothes and books, you're dealing with variables and coefficients. Intrigued? Let's explore further!

Throughout this article, we'll break down everything you need to know about standard form polynomial expressions. From understanding the basics to applying them in real-world scenarios, we've got all the juicy details for you. By the end of this, you'll be solving polynomial problems like a pro. Let's get to it!

Movierulz 2023 Ndash Your Ultimate Guide To Downloading Movies Safely And Legally

What Exactly is a Polynomial Expression?

Before we jump into the standard form, let's first wrap our heads around what a polynomial expression actually is. Simply put, a polynomial expression is a mathematical expression that consists of variables, coefficients, and constants combined using addition, subtraction, and multiplication. No division by variables allowed here, folks!

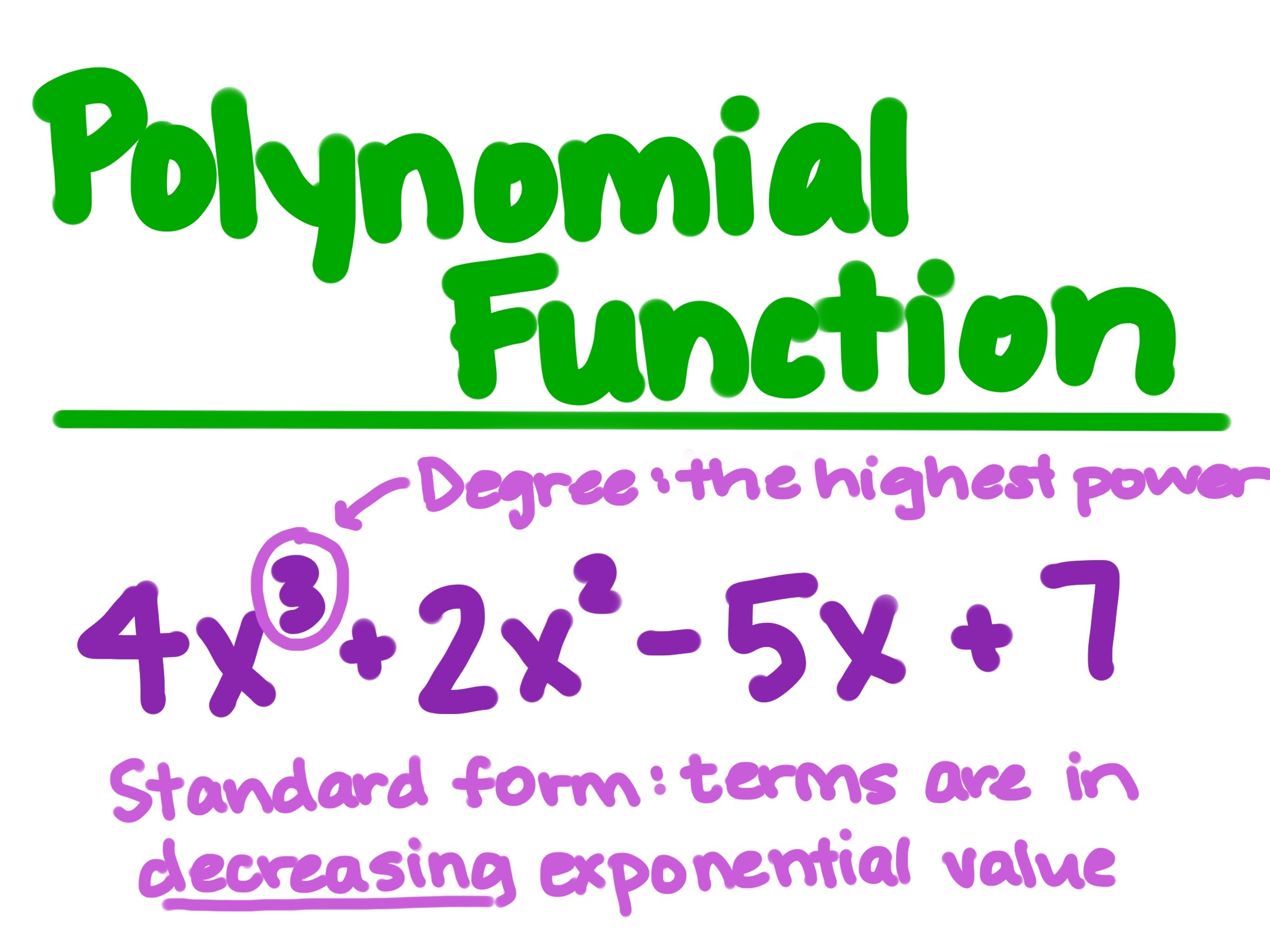
Polynomials can have one or more terms, and each term is made up of a variable raised to a non-negative integer power, multiplied by a coefficient. For example, 3x² + 2x - 5 is a polynomial expression with three terms. The degree of a polynomial is determined by the highest power of the variable in the expression. Cool, right?

The Importance of Standard Form

Now that we know what polynomials are, let's talk about why the standard form is so crucial. The standard form of a polynomial expression is when the terms are arranged in descending order of their degrees. This arrangement makes it easier to identify the degree of the polynomial and simplifies various mathematical operations.

Desipincom Your Ultimate Guide To The Rising Star In Digital Solutions

Imagine trying to solve a polynomial equation where the terms are all jumbled up. Sounds like a nightmare, doesn't it? By organizing the terms in standard form, we create a clear and structured framework for working with these expressions. It's like alphabetizing your books; everything falls into place, making it easier to navigate.

How to Write a Polynomial in Standard Form

Writing a polynomial in standard form is simpler than you might think. First, identify all the terms in the expression and determine the degree of each term. Then, arrange the terms in descending order based on their degrees. If there are like terms, combine them to simplify the expression.

For example, take the polynomial 4x³ + 9x - 2x² + 5. To write it in standard form, rearrange the terms as follows: 4x³ - 2x² + 9x + 5. See how much neater and more organized it looks? This format makes it a breeze to work with the polynomial in various mathematical contexts.

Common Mistakes to Avoid

Even the best of us make mistakes when working with polynomials. One common error is forgetting to arrange the terms in descending order of their degrees. Another pitfall is neglecting to combine like terms, which can lead to incorrect results.

To avoid these blunders, always double-check your work. Take your time to ensure that every term is in its rightful place and that no like terms are left uncombined. Remember, precision is key when dealing with polynomials. Don't rush the process; accuracy matters more than speed.

Applications of Standard Form Polynomial Expressions

So, why do we even bother with standard form polynomial expressions? Well, they have numerous applications in various fields. In engineering, polynomials are used to model physical phenomena, such as the motion of objects or the behavior of electrical circuits. In economics, they help analyze cost and revenue functions.

Even in everyday life, polynomials come into play. Ever calculated the area of a rectangular garden or figured out how much paint you need for a wall? Chances are, you used a polynomial expression to do so. Understanding standard form allows us to tackle these real-world problems with confidence.

Tips for Solving Polynomial Problems

Here are a few tips to help you conquer polynomial problems with ease:

Always write the polynomial in standard form before attempting any calculations.
Break down complex problems into smaller, more manageable steps.
Use visual aids, such as graphs or charts, to better understand the problem.
Practice, practice, practice! The more problems you solve, the more comfortable you'll become with polynomials.

Remember, becoming a polynomial expert doesn't happen overnight. It takes time, patience, and dedication. But with these tips in your arsenal, you'll be well on your way to mastering this essential math concept.

Advanced Concepts in Polynomial Expressions

Once you've mastered the basics, it's time to dive into some advanced concepts. One such concept is factoring polynomials, which involves breaking down a polynomial into simpler expressions that, when multiplied together, give the original polynomial. Factoring is a powerful tool for solving equations and simplifying expressions.

Another advanced topic is polynomial division, which allows us to divide one polynomial by another. This process is similar to long division in arithmetic and is crucial for solving higher-degree polynomial equations.

Real-World Examples of Polynomial Expressions

Let's take a look at some real-world examples where polynomial expressions come into play:

Physics: Polynomials are used to describe the motion of objects, such as the trajectory of a projectile.
Finance: They help model investment growth and calculate interest rates over time.
Computer Science: Polynomials are integral to algorithms for data compression and encryption.

These examples demonstrate the versatility and importance of polynomial expressions in various disciplines. By understanding standard form, you gain the ability to apply this knowledge across a wide range of fields.

Exploring Polynomial Functions

Polynomial functions are mathematical functions that can be expressed as polynomial expressions. They are widely used in calculus, where they play a crucial role in differentiation and integration. The graph of a polynomial function can reveal valuable information about its behavior, such as its roots, maxima, and minima.

Understanding polynomial functions is essential for anyone pursuing a career in mathematics, science, or engineering. They provide a solid foundation for more advanced mathematical concepts and applications.

Resources for Learning More About Polynomials

If you're eager to deepen your understanding of polynomials, there are plenty of resources available. Online platforms like Khan Academy and Coursera offer free courses and tutorials on algebra and polynomial expressions. Additionally, textbooks and academic papers provide in-depth insights into the theory and applications of polynomials.

Don't hesitate to explore these resources and expand your knowledge. The more you learn, the better equipped you'll be to tackle polynomial problems and apply this knowledge in real-world scenarios.

Conclusion: Take Action and Master Polynomials

And there you have it, folks! A comprehensive guide to standard form polynomial expressions. From understanding the basics to exploring advanced concepts, we've covered it all. Remember, mastering polynomials takes practice and perseverance, but the rewards are well worth the effort.

Now it's your turn to take action. Share this article with your friends, leave a comment with your thoughts, and check out our other articles for more math tips and tricks. Together, let's unlock the secrets of mathematics and conquer the world of polynomials!